Tailieumoi.vn xin giới thiệu đến các quý thầy cô, các em học sinh đang trong quá trình ôn tập tài liệu Chuyên đề phương trình và bất phương trình chứa căn, tài liệu bao gồm 26 trang. Tài liệu được tổng hợp từ các tài liệu ôn thi hay nhất  giúp các em học sinh có thêm tài liệu tham khảo trong quá trình ôn tập, củng cố kiến thức và chuẩn bị cho kỳ thi sắp hới. Chúc các em học sinh ôn tập thật hiệu quả và đạt được kết quả như mong đợi.

Mời các quý thầy cô và các em học sinh cùng tham khảo và tải về chi tiết tài liệu dưới đây

Chuyên đề phương trình và bất phương trình chứa căn

Chuyên đề 19: phươnng trình & bất phương trình chứa căn thức

I. Các kiến thức cơ bản

Người ta hay dùng các phương trình và bất phương trình chứa căn thức cơ bản sau đây:

\(*\sqrt {f(x)}  = g(x)(1).\) Ta có \((1) \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{f(x) \ge 0}\\{g(x) \ge 0}\\{f(x) = {g^2}(x)}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{g(x) \ge 0}\\{f(x) = {g^2}(x)}\end{array}} \right.} \right.\)

\(\begin{array}{l}*\sqrt {f(x)}  < g(x)(2).{\rm{ Ta co  }}(2) \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{f(x) \ge 0}\\{g(x) \ge 0}\\{f(x) < {g^2}(x)}\end{array}} \right.\\*\sqrt {f(x)}  > g(x)(3).{\rm{ Ta co  (3) }} \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{\left\{ {\begin{array}{*{20}{l}}{f(x) \ge 0}\\{g(x) < 0}\\{\{ g(x) \ge 0}\\{f(x) > {g^2}(x)}\end{array}} \right.}\end{array}} \right.\end{array}\)

II. Các dạng toán cơ bản

Loại 1. Phương trình và bất phương trình chứa căn thức cơ bản

Thí dụ 1:

Giải các phương trình sau:

\(1/\sqrt {6 - 4x + {x^2}}  = x + 4\)

2/ \(\sqrt {x + 5}  - \sqrt {x - 3}  = 2\)

\(3/\sqrt {3x + 4}  + \sqrt {x + 4}  = 2\sqrt x \)

Bài giải:

1/ Xét phương trình: \(\sqrt {6 - 4x + {x^2}}  = x + 4\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{6 - 4x + {x^2} \ge 0}\\{x + 4 \ge 0}\\{6 - 4x + {x^2} = {{(x + 4)}^2}}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge  - 4}\\{6 - 4x + {x^2} = {x^2} + 8x + 16}\end{array}} \right.} \right.\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge  - 4}\\{x =  - \frac{5}{6}}\end{array} \Leftrightarrow x =  - \frac{5}{6}} \right.\)

2/ Xét phương trình: \(\sqrt {x + 5}  - \sqrt {x - 3}  = 2\)

\( \Leftrightarrow \sqrt {x + 5}  = 2 + \sqrt {x - 3}  \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x + 5 \ge 0}\\{x - 3 \ge 0}\\{x + 5 = 4 + x - 3 + 4\sqrt {x - 3} }\end{array}} \right.\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge 3}\\{1 = \sqrt {x - 3} }\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge 3}\\{x - 3 = 1}\end{array} \Leftrightarrow x = 4} \right.} \right.\)

3. Xét phương trình \(3/\sqrt {3x + 4}  + \sqrt {x + 4}  = 2\sqrt x \)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{3x + 4 \ge 0}\\{x + 4 \ge 0}\\{x \ge 0}\\{3x + 4 + x + 4 + 2\sqrt {(3x + 4)(x + 4)}  = 4x}\end{array}} \right.\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge 0(1)}\\{4 + \sqrt {(3x + 4)(x + 4)}  = 0(2)}\end{array}} \right.\)

Rõ ràng \(VT(2) > 0\forall x \ge 0\). Vậy hệ (1) (2) vô nghiệm. Suy ra phương trình đã cho vô nghiệm.

Thí dụ 2:

Giải các phương trình sau:

1/ \(3{x^2} + 15x + 2\sqrt {{x^2} + 5x + 1}  = 2\)

2/ \(\sqrt {{x^2} + x + 4}  + \sqrt {{x^2} + x + 1}  = \sqrt {2{x^2} + 2x + 9} \)

3/\(\frac{4}{{x + \sqrt {{x^2} + x} }} - \frac{1}{{x - \sqrt {{x^2} + x} }} = \frac{3}{x}\)

4/\(\sqrt {x + 2\sqrt {x - 1} }  + \sqrt {x - 2\sqrt {x - 1} }  = 2\)

5/ \(\sqrt {x + 3 - 4\sqrt {x - 1} }  + \sqrt {x + 8 - 6\sqrt {x - 1} }  = 1\)

6/\(\sqrt[3]{{x + 1}} - \sqrt[3]{{x - 1}} = \sqrt[6]{{{x^2} - 1}}\)

Bài giải:

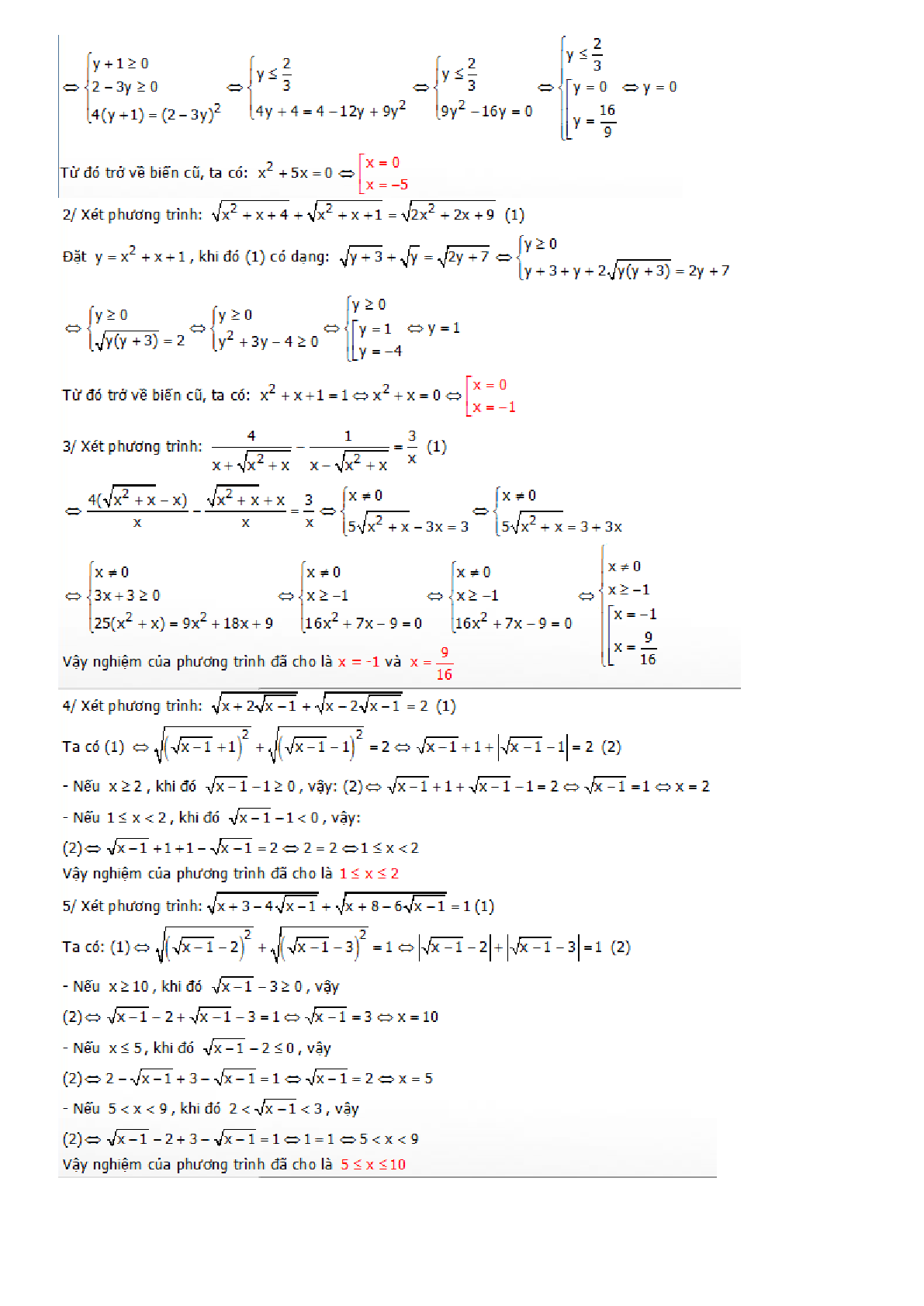
1/ Xét phương trình: \(3{x^2} + 15x + 2\sqrt {{x^2} + 5x + 1}  = 2\) (1)

Đặt \(y = {x^2} + 5x\), khi đó (1) có dạng: \(3y + 2\sqrt {y + 1}  = 2 \Leftrightarrow 2\sqrt {y + 1}  = 2 - 3y\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y + 1 \ge 0}\\{2 - 3y \ge 0}\\{4(y + 1) = {{(2 - 3y)}^2}}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \le \frac{2}{3}}\\{4y + 4 = 4 - 12y + 9{y^2}}\end{array}} \right.} \right.\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \le \frac{2}{3}}\\{9{y^2} - 16y = 0}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \le \frac{2}{3}}\\{y = 0}\\{y = \frac{{16}}{9}}\end{array} \Leftrightarrow y = 0} \right.} \right.\)

Từ đó trớ về biển cũ, ta có: \({x^2} + 5x = 0 \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{x = 0}\\{x =  - 5}\end{array}} \right.\)

2/ Xét phương trình: \(\sqrt {{x^2} + x + 4}  + \sqrt {{x^2} + x + 1}  = \sqrt {2{x^2} + 2x + 9} \) (1)

Đặt \(y = {x^2} + x + 1\), khi đó (1) có dạng:

\(\begin{array}{l}\sqrt {y + 3}  + \sqrt y  = \sqrt {2y + 7} \\ \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \ge 0}\\{y + 3 + y + 2\sqrt {y(y + 3)}  = 2y + 7}\end{array}} \right.\end{array}\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \ge 0}\\{\sqrt {y(y + 3)}  = 2}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \ge 0}\\{{y^2} + 3y - 4 \ge 0}\end{array}} \right.} \right.\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \ge 0}\\{\left[ {\begin{array}{*{20}{l}}{y = 1}\\{y =  - 4}\end{array}} \right.}\end{array} \Leftrightarrow y = 1} \right.\)

Từ đó trở về biến cũ, ta có:

\({x^2} + x + 1 = 1 \Leftrightarrow {x^2} + x = 0 \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{x = 0}\\{x =  - 1}\end{array}} \right.\)

3/ Xét phương trình: \(\frac{4}{{x + \sqrt {{x^2} + x} }} - \frac{1}{{x - \sqrt {{x^2} + x} }} = \frac{3}{x}\) (1)

\(\begin{array}{l} \Leftrightarrow \frac{{4\left( {\sqrt {{x^2} + x}  - x} \right)}}{x} - \frac{{\sqrt {{x^2} + x}  + x}}{x} = \frac{3}{x}\\ \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ne 0}\\{5\sqrt {{x^2} + x}  - 3x = 3}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ne 0}\\{5\sqrt {{x^2} + x}  = 3 + 3x}\end{array}} \right.} \right.\end{array}\)

\[ \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ne 0}\\{3x + 3 \ge 0}\\{25\left( {{x^2} + x} \right) = 9{x^2} + 18x + 9}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ne 0}\\{x \ge  - 1}\\{16{x^2} + 7x - 9 = 0}\end{array}} \right.} \right.\]

\[ \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ne 0}\\{x \ge  - 1}\\{16{x^2} + 7x - 9 = 0}\end{array}} \right. \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ne 0}\\{x \ge  - 1}\\{\left[ {\begin{array}{*{20}{c}}{x =  - 1}\\{x = \frac{9}{{16}}}\end{array}} \right.}\end{array}} \right.\]

Vậy nghiệm của phương trình đã cho là \(x =  - 1\) và \(x = \frac{9}{{16}}\)

4/ Xét phương trình: \(\sqrt {x + 2\sqrt {x - 1} }  + \sqrt {x - 2\sqrt {x - 1} }  = 2\) (1)

Ta có \((1) \Leftrightarrow \sqrt {{{(\sqrt {x - 1}  + 1)}^2}}  + \sqrt {{{(\sqrt {x - 1}  - 1)}^2}}  = 2\)

\( \Leftrightarrow \sqrt {x - 1}  + 1 + |\sqrt {x - 1}  - 1| = 2\) (2)

- Nếu \(x \ge 2\), khi đó \(\sqrt {x - 1}  - 1 \ge 0\), vậy: (2) \( \Leftrightarrow \sqrt {x - 1}  + 1 + \sqrt {x - 1}  - 1 = 2 \Leftrightarrow \sqrt {x - 1}  = 1 \Leftrightarrow x = 2\)

- Nếu \(1 \le x < 2\), khi đó \(\sqrt {x - 1}  - 1 < 0\), vậy:

(2) \( \Leftrightarrow \sqrt {x - 1}  + 1 + 1 - \sqrt {x - 1}  = 2 \Leftrightarrow 2 = 2 \Leftrightarrow 1 \le x < 2\)

Vậy nghiệm cúa phương trình đã cho là \(1 \le x \le 2\)

5/ Xét phương trình: \(\sqrt {x + 3 - 4\sqrt {x - 1} }  + \sqrt {x + 8 - 6\sqrt {x - 1} }  = 1(1)\)

Ta có: \((1) \Leftrightarrow \sqrt {{{(\sqrt {x - 1}  - 2)}^2}}  + \sqrt {{{(\sqrt {x - 1}  - 3)}^2}}  = 1\)

\( \Leftrightarrow |\sqrt {x - 1}  - 2| + |\sqrt {x - 1}  - 3| = 1\) (2)

- Nếu \(x \ge 10\), khi đó \(\sqrt {x - 1}  - 3 \ge 0\), vậy

\((2) \Leftrightarrow \sqrt {x - 1}  - 2 + \sqrt {x - 1}  - 3 = 1 \Leftrightarrow \sqrt {x - 1}  = 3 \Leftrightarrow x = 10\)

- Nếu \(x \le 5\), khi đó \(\sqrt {x - 1}  - 2 \le 0\), vậy

(2) \( \Leftrightarrow 2 - \sqrt {x - 1}  + 3 - \sqrt {x - 1}  = 1 \Leftrightarrow \sqrt {x - 1}  = 2 \Leftrightarrow x = 5\)

- Nếu \(5 < x < 9\), khi đó \(2 < \sqrt {x - 1}  < 3\), vậy

( 2\() \Leftrightarrow \sqrt {x - 1}  - 2 + 3 - \sqrt {x - 1}  = 1 \Leftrightarrow 1 = 1 \Leftrightarrow 5 < x < 9\)

Vậy nghiệm cúa phương trình đã cho là \(5 \le x \le 10\)

6/ Xét phương trình: \(\sqrt[3]{{x + 1}} - \sqrt[3]{{x - 1}} = \sqrt[6]{{{x^2} - 1}}\) (1)

Điều kiện là: \({x^2} - 1 \ge 0 \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{x \ge 1}\\{x \le  - 1}\end{array}} \right.\)

Do nễu \({x_0} \ge 1\) là nghiệm cúa (1) thì \( - {x_0}\) cũng là nghiệm của (1)

Vì thế tạm xét (1) với \(x \ge 1\)

Khi \(x = 1\), thì \({\rm{VP}}(1) = 0;\quad VT(1) \ne 0 \Rightarrow x = 1\) không phải là nghiệm.

Khi \(x > 1\), thi \(\sqrt[6]{{{x^2} - 1}} > 0\)

Vậy \((1) \Leftrightarrow \sqrt[6]{{\frac{{{{(x + 1)}^2}}}{{{x^2} - 1}}}} - 6\sqrt[6]{{\frac{{{{(x - 1)}^2}}}{{{x^2} - 1}}}} = 1 \Leftrightarrow 6\sqrt {\frac{{x + 1}}{{x - 1}}}  - 6\sqrt[6]{{\frac{{x - 1}}{{x + 1}}}} - 1 = 0\) (2)

Đặt \(y = \frac{6}{{\frac{{x + 1}}{{x - 1}}}} > 0\), thì (2) có dạng:

 \(y - \frac{1}{y} - 1 = 0 \Leftrightarrow {y^2} - y - 1 = 0 \Leftrightarrow y = \frac{{1 + \sqrt 5 }}{2}\) (do y>0)

\( \Leftrightarrow \sqrt[6]{{\frac{{x + 1}}{{x - 1}}}} = \frac{{1 + \sqrt 5 }}{2} \Leftrightarrow \frac{{x + 1}}{{x - 1}} = {k^6}\) (ở đây \(k = \frac{{1 + \sqrt 5 }}{2}\) )

\( \Leftrightarrow 1 + \frac{2}{{x - 1}} = {k^6} \Leftrightarrow \frac{2}{{x - 1}} = {k^6} - 1 \Leftrightarrow x = \frac{{{k^6} + 1}}{{{k^6} - 1}}\)

Vậy phương trình đã cho có hai nghiệm là \(x =  \pm \frac{{{k^6} + 1}}{{{k^6} - 1}}\), với \(k = \frac{{1 + \sqrt 5 }}{2}\)

Thí dụ 3:

Giải các bất phương trình sau:

1/ \(\sqrt {{x^2} + 3x + 3}  < 2x + 1\)

\(2/\sqrt {8 + 2x - {x^2}}  > 6 - 3x\)

\(3/\sqrt {x + 3}  + \sqrt {x + 2}  - \sqrt {2x + 4}  > 0\)

\(4/\sqrt {3{x^2} + 5x + 7}  - \sqrt {3{x^2} + 5x + 2}  > 1\)

Bài giải:

1/ Xét bất phương trình \(\sqrt {{x^2} + 3x + 3}  < 2x + 1\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{{x^2} + 3x + 3 \ge 0}\\{2x + 1 \ge 0}\\{{x^2} + 3x + 3 < {{(2x + 1)}^2}}\end{array}} \right.\)

Do \({x^2} + 3x + 3 > 0\forall x(\) vì \(\Delta  = 9 - 12 < 0)\) nên: (1) (2) (3) \(\left\{ {\begin{array}{*{20}{l}}{x \ge  - \frac{1}{2}}\\{3{x^2} + x - 2 > 0}\end{array} \Leftrightarrow x > \frac{2}{3}} \right.\)

2/ Xét bất phương trình \(\sqrt {8 + 2x - {x^2}}  > 6 - 3x(1)\)

Ta có (1)

\( \Rightarrow \left[ {\begin{array}{*{20}{l}}{\left\{ {\begin{array}{*{20}{l}}{8 + 2x - {x^2} \ge 0}\\{6 - 3x < 0}\end{array}} \right.}\\{\left\{ {\begin{array}{*{20}{l}}{8 + 2x - {x^2} \ge 0}\\{6 - 3x \ge 0}\\{8 + 2x - {x^2} > {{(6 - 3x)}^2}}\end{array}} \right.}\end{array}} \right. \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{\left\{ {\begin{array}{*{20}{c}}{ - 2 \le x \le 4}\\{x > 2}\end{array}} \right.}\\{\left\{ {\begin{array}{*{20}{c}}{x \le 2}\\{5{x^2} - 19x + 14 < 0}\end{array}} \right.}\end{array}} \right.\)

\( \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{2 < x \le 4}\\{1 < x \le 2}\end{array} \Leftrightarrow 1 < x \le 4} \right.\)

3/ Xét bất phương trình \(\sqrt {x + 3}  + \sqrt {x + 2}  - \sqrt {2x + 4}  > 0\)

\(\begin{array}{l} \Leftrightarrow \sqrt {x + 3}  + \sqrt {x + 2}  > \sqrt {2x + 4} \\ \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge  - 2}\\{(x + 3) + (x + 2) + 2\sqrt {(x + 3)(x + 2)}  > 2x + 4}\end{array}} \right.\end{array}\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge  - 2}\\{1 + 2\sqrt {(x + 3)(x + 2)}  > 0}\end{array} \Leftrightarrow x \ge  - 2} \right.\)

4/ Xét bất phương trình \(\sqrt {3{x^2} + 5x + 7}  - \sqrt {3{x^2} + 5x + 2}  > 1\)

Đặt \(y = 3{x^2} + 5x + 2\), khi đó từ \((1)\) có \(\sqrt {y + 2}  - \sqrt y  > 1 \Leftrightarrow \sqrt {y + 2}  > 1 + \sqrt y \)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \ge 0}\\{y + 2 > 1 + y + 2\sqrt y }\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \ge 0}\\{1 > 2\sqrt y }\end{array} \Leftrightarrow 0 \le y \le \frac{1}{4}} \right.} \right.\)

Trở về biến cũ, ta có hệ sau: \(\left\{ {\begin{array}{*{20}{l}}{3{x^2} + 5x + 2 \ge 0}\\{3{x^2} + 5x + 2 \le \frac{1}{4}}\end{array}} \right.\)

Biểu diễn trên trục sồ ta có:

Từ đó suy ra nghiệm cần tìm là: \( - \frac{7}{6} \le x \le  - 1\)

Thí dụ 4:

Giải các bất phương trình sau:

\(1/\frac{{1 - \sqrt {1 - 4{x^2}} }}{x} < 3\)

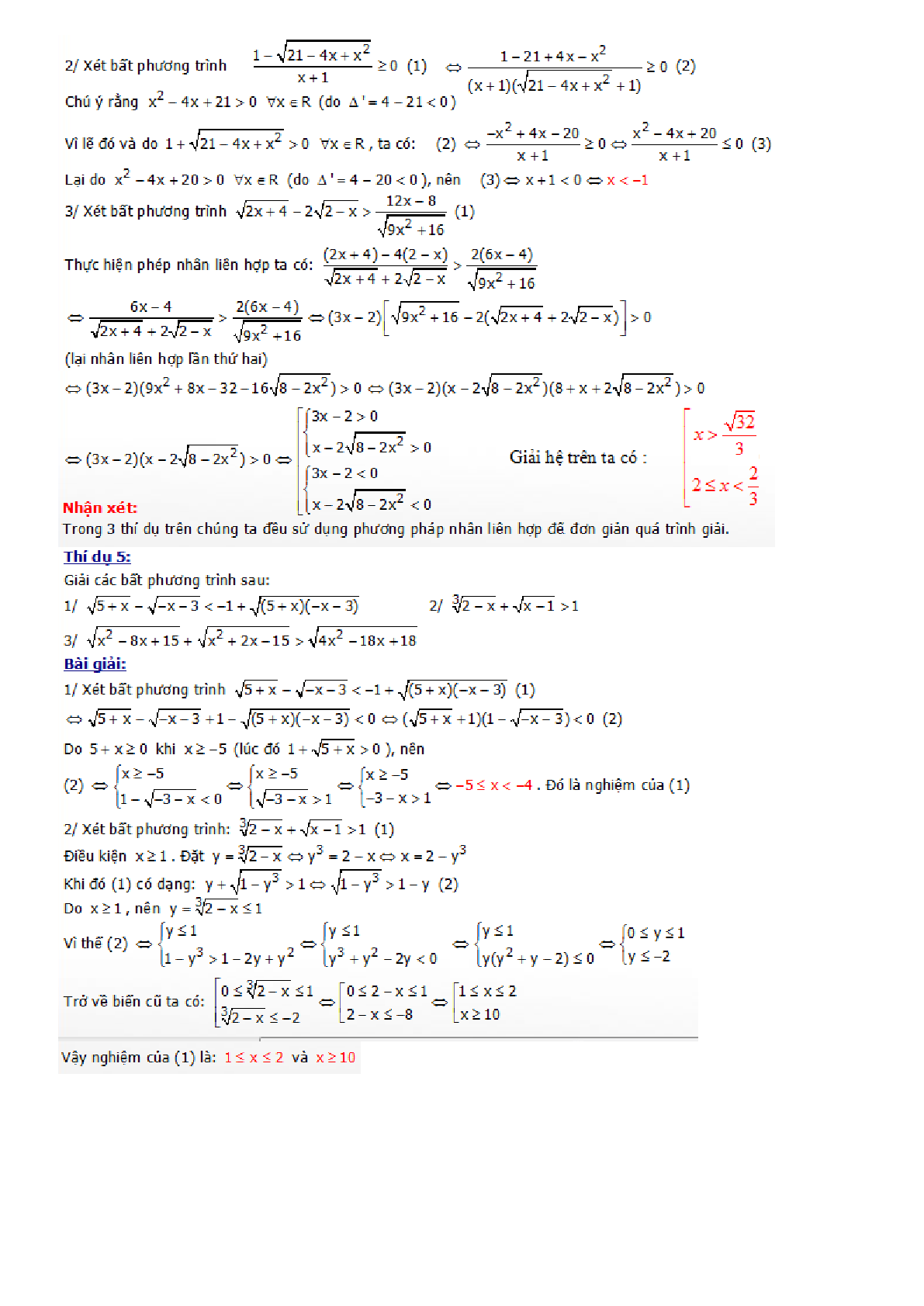
\(2/\frac{{1 - \sqrt {21 - 4x + {x^2}} }}{{x + 1}} \ge 0\)

\(3/\sqrt {2x + 4}  - 2\sqrt {2 - x}  > \frac{{12x - 8}}{{\sqrt {9{x^2} + 16} }}\)

Bài giải:

\(1/\) Xét bất phương trình \(\frac{{1 - \sqrt {1 - 4{x^2}} }}{x} < 3(1)\)

Chú ý \(x \ne 0\) và dùng phép nhân liên hợp ta có:

\((1) \Leftrightarrow \left\{ {\frac{{\left( {1 - \sqrt {1 - 4{x^2}} } \right)\left( {1 + \sqrt {1 - 4{x^2}} } \right)}}{{x\left( {1 + \sqrt {1 - 4{x^2}} } \right)}} < 3} \right.\) \(\quad (x \ne 0\)

\(\left[ {x\left( {1 + \sqrt {1 - 4{x^2}} } \right) \cdot \left[ {\begin{array}{*{20}{l}}{x \ne 0}\\{1 + 4{x^2} \ge 0}\end{array} - \frac{1}{2} \le x \le \frac{1}{2}} \right.} \right.\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ne 0}\\{4x - 3 < 3\sqrt {1 - 4{x^2}} }\end{array} \Leftrightarrow \begin{array}{*{20}{l}}{1 - 4{x^2} \ge 0}\\{4x - 3 < 0}\\{x \ne 0}\end{array}} \right.\)

(do \(1 + \sqrt {1 - 4{x^2}}  > 0\) khi có nghiệm)

vì hệ \(\left\{ {x \ge \frac{3}{4}} \right.\)

$\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge \frac{3}{4}}\\{0 < x < \frac{6}{{13}}}\end{array}} \right.\)

Chú ý rằng \({x^2} - 4x + 21 > 0\forall x \in R\) (do \({\Delta ^\prime } = 4 - 21 < 0\) )

Vì lẽ đó và do \(1 + \sqrt {21 - 4x + {x^2}}  > 0\quad \forall x \in R\), ta có: \(\quad (2) \Leftrightarrow \frac{{ - {x^2} + 4x - 20}}{{x + 1}} \ge 0 \Leftrightarrow \frac{{{x^2} - 4x + 20}}{{x + 1}} \le 0\) (3)

Lại do \({x^2} - 4x + 20 > 0\quad \forall x \in R\) (do \({\Delta ^\prime } = 4 - 20 < 0\) ), nên \(\quad (3) \Leftrightarrow x + 1 < 0 \Leftrightarrow x <  - 1\)

3/ Xét bẫt phương trình \(\sqrt {2x + 4}  - 2\sqrt {2 - x}  > \frac{{12x - 8}}{{\sqrt {9{x^2} + 16} }}\) (1)

Thực hiện phép nhân liên hợp ta có: \(\frac{{(2x + 4) - 4(2 - x)}}{{\sqrt {2x + 4}  + 2\sqrt {2 - x} }} > \frac{{2(6x - 4)}}{{\sqrt {9{x^2} + 16} }}\)

\( \Leftrightarrow \frac{{6x - 4}}{{\sqrt {2x + 4}  + 2\sqrt {2 - x} }} > \frac{{2(6x - 4)}}{{\sqrt {9{x^2} + 16} }} \Leftrightarrow (3x - 2)\left[ {\sqrt {9{x^2} + 16}  - 2(\sqrt {2x + 4}  + 2\sqrt {2 - x} )} \right] > 0\)

(lại nhân liên hợp lần thứ hai)

Troạng 3 thí dụ trên chúng ta đều sử dụng phương pháp nhân liên hợp đẽ đơn giản quá trình giải.

Thí du 5:

Giải các bất phương trình sau:

1/ \(\sqrt {5 + x}  - \sqrt { - x - 3}  <  - 1 + \sqrt {(5 + x)( - x - 3)} \)

\(2/\sqrt[3]{{2 - x}} + \sqrt {x - 1}  > 1\)

3/ \(\sqrt {{x^2} - 8x + 15}  + \sqrt {{x^2} + 2x - 15}  > \sqrt {4{x^2} - 18x + 18} \)

Bài giải:

1/ Xét bẫt phương trình \(\sqrt {5 + x}  - \sqrt { - x - 3}  <  - 1 + \sqrt {(5 + x)( - x - 3)} \) (1)

\(\begin{array}{l} \Leftrightarrow \sqrt {5 + x}  - \sqrt { - x - 3}  + 1 - \sqrt {(5 + x)( - x - 3)}  < 0\\ \Leftrightarrow (\sqrt {5 + x}  + 1)(1 - \sqrt { - x - 3} ) < 0\;\left( 2 \right)\end{array}\)

Do \(5 + x \ge 0\) khi \(x \ge  - 5\) (lúc đó \(1 + \sqrt {5 + x}  > 0\) ), nên

(2) \[ \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge  - 5}\\{1 - \sqrt { - 3 - x}  < 0}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge  - 5}\\{\sqrt { - 3 - x}  > 1}\end{array}} \right.} \right.\]

\[ \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{x \ge  - 5}\\{ - 3 - x > 1}\end{array} \Leftrightarrow  - 5 \le x <  - 4} \right.\].

 Đó là nghiệm của (1)

2/ Xét bẫt phương trình: \(\sqrt[3]{{2 - x}} + \sqrt {x - 1}  > 1\) (1)

Điều kiện \(x \ge 1\). Đặt \(y = \sqrt[3]{{2 - x}} \Leftrightarrow {y^3} = 2 - x \Leftrightarrow x = 2 - {y^3}\)

Khi đó (1) có dạng: \(y + \sqrt {1 - {y^3}}  > 1 \Leftrightarrow \sqrt {1 - {y^3}}  > 1 - y\) (2)

Do \(x \ge 1\), nên \(y = \sqrt[3]{{2 - x}} \le 1\)

Vĩ thẽ \((2) \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \le 1}\\{1 - {y^3} > 1 - 2y + {y^2}}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \le 1}\\{{y^3} + {y^2} - 2y < 0}\end{array}} \right.} \right.\)

\( \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{y \le 1}\\{y\left( {{y^2} + y - 2} \right) \le 0}\end{array} \Leftrightarrow \left\{ {\begin{array}{*{20}{l}}{0 \le y \le 1}\\{y \le  - 2}\end{array}} \right.} \right.\)

Trở về biển cũ ta có: \(\left[ {\begin{array}{*{20}{l}}{0 \le \sqrt[3]{{2 - x}} \le 1}\\{\sqrt[3]{{2 - x}} \le  - 2}\end{array} \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{0 \le 2 - x \le 1}\\{2 - x \le  - 8}\end{array} \Leftrightarrow \left[ {\begin{array}{*{20}{l}}{1 \le x \le 2}\\{x \ge 10}\end{array}} \right.} \right.} \right.\)

Vậy nghiệm cúa (1) là: \(1 \le x \le 2\) và \(x \ge 10\)